Our website is made possible by displaying online advertisements to our visitors.
Please consider supporting us by disabling your ad blocker.
Symmetric group
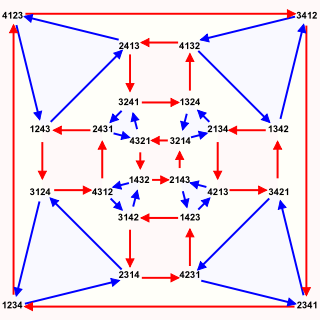

These are the positions of the six matrices
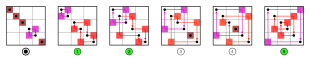
Some matrices are not arranged symmetrically to the main diagonal – thus the symmetric group is not abelian.
| Algebraic structure → Group theory Group theory |
|---|
 |
In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. In particular, the finite symmetric group defined over a finite set of symbols consists of the permutations that can be performed on the symbols.[1] Since there are ( factorial) such permutation operations, the order (number of elements) of the symmetric group is .
Although symmetric groups can be defined on infinite sets, this article focuses on the finite symmetric groups: their applications, their elements, their conjugacy classes, a finite presentation, their subgroups, their automorphism groups, and their representation theory. For the remainder of this article, "symmetric group" will mean a symmetric group on a finite set.
The symmetric group is important to diverse areas of mathematics such as Galois theory, invariant theory, the representation theory of Lie groups, and combinatorics. Cayley's theorem states that every group is isomorphic to a subgroup of the symmetric group on (the underlying set of) .
- ^ Jacobson 2009, p. 31
Previous Page Next Page







