Our website is made possible by displaying online advertisements to our visitors.
Please consider supporting us by disabling your ad blocker.
Integral
Formula:
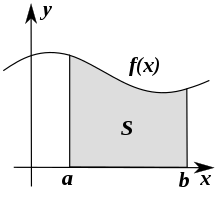
In calculus, an integral is the space under a graph of an equation (sometimes said as "the area under a curve"). An integral is the reverse of a derivative, and integral calculus is the opposite of differential calculus. A derivative is the steepness (or "slope"), as the rate of change, of a curve. The word "integral" can also be used as an adjective meaning "related to integers".
The symbol for integration, in calculus, is: as a tall letter "S".[1][2][3]
Integrals and derivatives are part of a branch of mathematics called calculus. The link between these two is very important, and is called the fundamental theorem of calculus.[4] The theorem says that an integral can be reversed by a derivative, similar to how an addition can be reversed by a subtraction.
Integration helps when trying to multiply units into a problem. For example, if a problem with rate, , needs an answer with just distance, one solution is to integrate with respect to time. This means multiplying in time to cancel the time in . This is done by adding small slices of the rate graph together. The slices are close to zero in width, but adding them together indefinitely makes them add up to a whole. This is called a Riemann sum.
Adding these slices together gives the equation that the first equation is the derivative of. Integrals are like a way to add many tiny things together by hand. It is like summation, which is adding . The difference with integration is that we also have to add all the decimals and fractions in between.[4]
Another time integration is helpful is when finding the volume of a solid. It can add two-dimensional (without width) slices of the solid together indefinitely—until there is a width. This means the object now has three dimensions: the original two and a width. This gives the volume of the three-dimensional object described.
- ↑ "List of Calculus and Analysis Symbols". Math Vault. 2020-05-11. Retrieved 2020-09-18.
- ↑ Weisstein, Eric W. "Integral". mathworld.wolfram.com. Retrieved 2020-09-18.
- ↑ "Integral calculus - Encyclopedia of Mathematics". encyclopediaofmath.org. Retrieved 2020-09-18.
- ↑ 4.0 4.1 Barton, David; Stuart Laird (2003). "16". Delta Mathematics. Pearson Education. ISBN 0-582-54539-0.
Previous Page Next Page







