
Back Espai corbat Catalan Zakřivený prostor Czech Raumkrümmung German Spás cuarach GA מרחב עקום HE 구부러진 공간 Korean Закривен простор MK Gekromde ruimte Dutch Krökt rum Swedish 弯曲空间 Chinese
| Part of a series on |
| Spacetime |
|---|
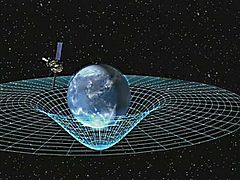 |
Curved space often refers to a spatial geometry which is not "flat", where a flat space has zero curvature, as described by Euclidean geometry.[1] Curved spaces can generally be described by Riemannian geometry, though some simple cases can be described in other ways. Curved spaces play an essential role in general relativity, where gravity is often visualized as curved spacetime.[2] The Friedmann–Lemaître–Robertson–Walker metric is a curved metric which forms the current foundation for the description of the expansion of the universe and the shape of the universe.[citation needed] The fact that photons have no mass yet are distorted by gravity, means that the explanation would have to be something besides photonic mass. Hence, the belief that large bodies curve space and so light, traveling on the curved space will, appear as being subject to gravity. It is not, but it is subject to the curvature of space.
- ^ "The Feynman Lectures on Physics Vol. II Ch. 42: Curved Space". www.feynmanlectures.caltech.edu. Retrieved 2024-01-18.
- ^ "Curved Space". www.math.brown.edu. Retrieved 2024-01-18.