
Back Demping AF تخميد Arabic Dempferləmə (söndürmə) AZ Esmorteïment Catalan Tlumené kmitání Czech Сÿнекен сулланусем CV Gwanychiad CY Dæmpning Danish Amortizo EO Amortiguamiento Spanish
| Part of a series on |
| Classical mechanics |
|---|
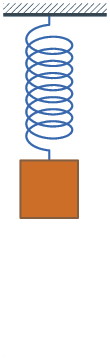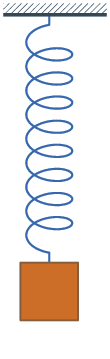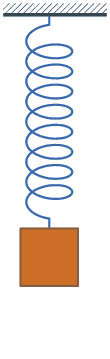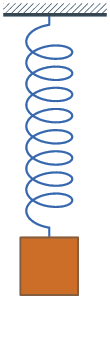
In physical systems, damping is the loss of energy of an oscillating system by dissipation.[1][2] Damping is an influence within or upon an oscillatory system that has the effect of reducing or preventing its oscillation.[3] Examples of damping include viscous damping in a fluid (see viscous drag), surface friction, radiation,[1] resistance in electronic oscillators, and absorption and scattering of light in optical oscillators. Damping not based on energy loss can be important in other oscillating systems such as those that occur in biological systems and bikes[4] (ex. Suspension (mechanics)). Damping is not to be confused with friction, which is a type of dissipative force acting on a system. Friction can cause or be a factor of damping.
The damping ratio is a dimensionless measure describing how oscillations in a system decay after a disturbance. Many systems exhibit oscillatory behavior when they are disturbed from their position of static equilibrium. A mass suspended from a spring, for example, might, if pulled and released, bounce up and down. On each bounce, the system tends to return to its equilibrium position, but overshoots it. Sometimes losses (e.g. frictional) damp the system and can cause the oscillations to gradually decay in amplitude towards zero or attenuate. The damping ratio is a measure describing how rapidly the oscillations decay from one bounce to the next.
The damping ratio is a system parameter, denoted by ζ ("zeta"), that can vary from undamped (ζ = 0), underdamped (ζ < 1) through critically damped (ζ = 1) to overdamped (ζ > 1).
The behaviour of oscillating systems is often of interest in a diverse range of disciplines that include control engineering, chemical engineering, mechanical engineering, structural engineering, and electrical engineering. The physical quantity that is oscillating varies greatly, and could be the swaying of a tall building in the wind, or the speed of an electric motor, but a normalised, or non-dimensionalised approach can be convenient in describing common aspects of behavior.
- ^ a b Escudier, Marcel; Atkins, Tony (2019). "A Dictionary of Mechanical Engineering". Oxford Reference. doi:10.1093/acref/9780198832102.001.0001. ISBN 978-0-19-883210-2.
- ^ Steidel (1971). An Introduction to Mechanical Vibrations. John Wiley & Sons. p. 37.
damped, which is the term used in the study of vibration to denote a dissipation of energy
- ^ Crandall, S. H. (January 1970). "The role of damping in vibration theory". Journal of Sound and Vibration. 11 (1): 3–18, IN1. Bibcode:1970JSV....11....3C. doi:10.1016/s0022-460x(70)80105-5.
- ^ J. P. Meijaard; J. M. Papadopoulos; A. Ruina & A. L. Schwab (2007). "Linearized dynamics equations for the balance and steer of a bicycle: a benchmark and review". Proceedings of the Royal Society A. 463 (2084): 1955–1982. Bibcode:2007RSPSA.463.1955M. doi:10.1098/rspa.2007.1857. S2CID 18309860.
lean and steer perturbations die away in a seemingly damped fashion. However, the system has no true damping and conserves energy. The energy in the lean and steer oscillations is transferred to the forward speed rather than being dissipated.
