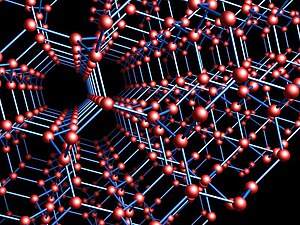
In geometry and crystallography, the Laves graph is an infinite and highly symmetric system of points and line segments in three-dimensional Euclidean space, forming a periodic graph. Three equal-length segments meet at 120° angles at each point, and all cycles use ten or more segments. It is the shortest possible triply periodic graph, relative to the volume of its fundamental domain. One arrangement of the Laves graph uses one out of every eight of the points in the integer lattice as its points, and connects all pairs of these points that are nearest neighbors, at distance . It can also be defined, divorced from its geometry, as an abstract undirected graph, a covering graph of the complete graph on four vertices.
H. S. M. Coxeter (1955) named this graph after Fritz Laves, who first wrote about it as a crystal structure in 1932.[1][2] It has also been called the K4 crystal,[3] (10,3)-a network,[4] diamond twin,[5] triamond,[6][7] and the srs net.[8] The regions of space nearest each vertex of the graph are congruent 17-sided polyhedra that tile space. Its edges lie on diagonals of the regular skew polyhedron, a surface with six squares meeting at each integer point of space.
Several crystalline chemicals have known or predicted structures in the form of the Laves graph. Thickening the edges of the Laves graph to cylinders produces a related minimal surface, the gyroid, which appears physically in certain soap film structures and in the wings of butterflies.
- ^ Cite error: The named reference
coxeterwas invoked but never defined (see the help page). - ^ Cite error: The named reference
laveswas invoked but never defined (see the help page). - ^ Cite error: The named reference
prl09was invoked but never defined (see the help page). - ^ Cite error: The named reference
wellswas invoked but never defined (see the help page). - ^ Cite error: The named reference
tlnkkwas invoked but never defined (see the help page). - ^ Cite error: The named reference
lanierwas invoked but never defined (see the help page). - ^ Cite error: The named reference
sequinwas invoked but never defined (see the help page). - ^ Cite error: The named reference
doywas invoked but never defined (see the help page).

